The Gömböc

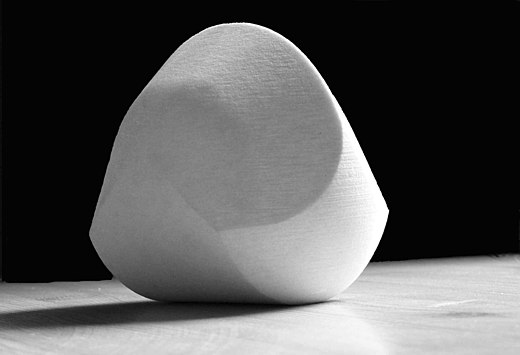
Gömböc in the Stable Equilibrium Position, Wikipedia

The Gömböc is described as “the shape that shouldn’t exist”. We learned more about it by watching this Youtube video by The Action Lab, which explained that this is the only known homogenous shape to have just 2 equilibrium points; one stable and one unstable. The cool thing about the Gömböc is that it is “self-righting” and has uniform density, so we thought it’d be fascinating to explore the math behind this shape by trying to make our very own Gömböc.

This is a challenging project because, on the one hand, this shape has uniform density, making it hard to re-create using a 3D Printer, whereas on the other hand, the very precise measurements and shape it has makes it difficult to construct out of uniformly dense material such as aluminum in the machine shop. Therefore, we’d be taking this project on with high risks of failure, but hoping to learn the enlightening mathematical truths behind the Gömböc through the making process. One way we were thinking of doing this, tackling the specific measurements and uniform density challenges all at once, is by creating a mold for it using the 3D printer, and then using a variety of materials to actually create the Gömböc. We found this file of a Gömböc 3D model we could potentially use for this.

The main mathematical concepts we’d be exploring in this project are what points of equilibrium are (including stable and unstable points), how we can find these theoretically, and understanding the math and physics behind monostatic shapes.

Tensegrity Structures

Dual Tensegrity, @M4NU from Printables.com

Since the very beginning of class, we’ve both been very intrigued by tensegrity structures. They take advantage of TENSional intEGRITY (hence the name) to create the illusion that things are floating in space. This project has a lot of mathematical and physical concepts we could explore, such as tension, compression, the mathematical modeling of tensegrity, and even things like measuring the “amount” of tensegrity in a particular structure.

There are many paths we could take to make this structure, ranging from 3D printing certain aspects (which we could do using this model), machine-shop the structure parts from either wood or metal, LEGOs, or even make it out of something more rudimentary like popsicle sticks (this video by Creative Fest is a nice tutorial for that)! Given that we only have 1 week to do project 0A, we’d probably opt for one of the less labor-intensive ones so that we can create a finished structure.

This would be a fun project because not only would it give us experience using machines we haven’t used before, but it would also give us an end-product that we can use to decorate our rooms!

Creating Sphericon Art

Quantum Sphericons, Altair3D on Etsy

Sphericons are a concept we stumbled upon while going down a Gömböc rabbit hole. Wikipedia defines them as “solid[s] that [have] a continuous developable surface with two congruent, semi-circular edges, and four vertices that define a square”. The Action Lab has a slightly more interesting way of describing them, as “The Shape That Meanders Instead of Rolls” (you should watch the video!)

As a project, we are really interested in not only creating a variety of sphericons, but also exploring their unique meandering paths. It’s almost like a research project investigating how different 3D sphericons translate into 2D art. We’d achieve this by dipping each sphericon in point, and “rolling” them down a canvas, varying our medium and methodology to create different pieces of Sphericon art. To make the sphericons themselves we’d use different techniques, like using the 3D printer and machine shop, and creating Sphericons using different types of coins (and other materials).

The mathematical concepts we’d be exploring with this project are the physics behind the sphericons’ meandering paths (and rollers in general) as well as their geometric properties, including continuous developable surfaces, surface areas, and volumes.