For Project 0.A, we decided to create art with a simple sphericon. Sphericons are defined as shapes that are obtained by “rotating a symmetric polygon about a mirror axis to get a solid of revolution, cutting that solid into two equal pieces, and then putting the pieces back together differently” (MOMATH). For this project, we decided to create a basic sphericon using just two coins. We then dipped these sphericons in acrylic paint, and rolled them down a sheet of paper in order to create art. Below, we outline the creative process in more detail, some of the challenges we encountered, and dive into the math of sphericons!

Creating our Sphericoins

To create our sphericons we used a variety of different coins. Doing the same with pennies, dimes and quarters, we utilized a Dremel with a serrated saw blade in order to cut a straight line into the center of each coin. Once we had done this for two separate coins of the same size, we proceeded to hammer the two coins together through their cut sides, as pictured below;

Once the coins were lodged together, and couldn’t be moved by our fingers alone, we had a stable sphericon made out of coins – or as we like to call it, a sphericoin! We then proceeded to repeat this process with 3 different coin types; pennies, dimes and quarters.

Meandering Art

One of the really cool properties of sphericons is that, as the Action Lab states, they are shapes which’s geometry causes them to “meander instead of roll”. With this in mind, we wanted to create some artistic work with our Sphericoins such that we could capture this meandering path. In order to do this, we dipped our Sphericoins in different colors of acrylic paint, and let them roll down a canvas on a slight incline. When diping the Sphericoins in paint, we made sure to only cover the coins edges such that only the path traced by the coin’s edge would be visible on the final product.

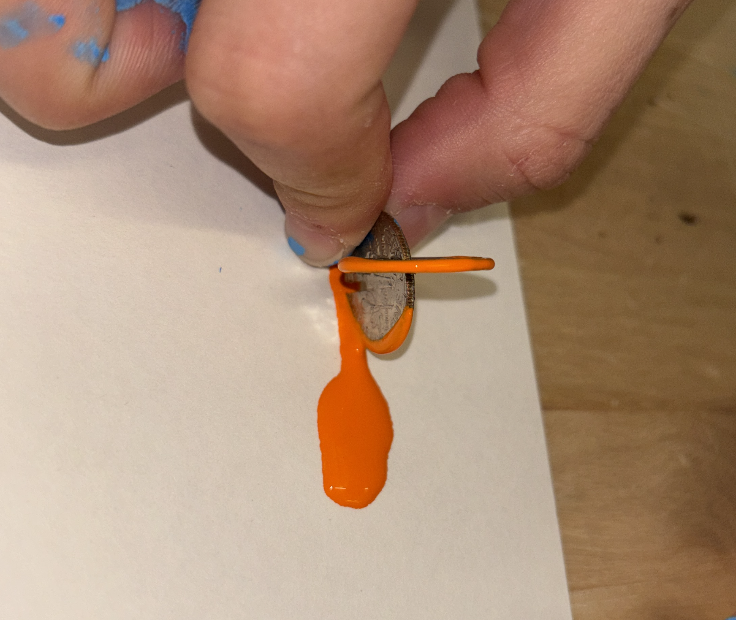
Once the coins were dipped in a thick layer of paint, we quickly rolled them down the canvas in order to try and capture as much of the Sphericoins’ paths as possible. We made sure to vary the size, initial conditions, and paint color of our Sphericoins in order to get interesting patterns! Our final product can be seen below;

Surprising Challenges

When we began trying to figure out how we could cut through a coin, we encountered some obstacles. Initially, we thought we could use Tin Snips to cut our coins. However, after trying this on a few different coins, we realized this would prevent us from actually attaching two coins together. The Tin Snips were able to cut a coin, but they also heavily bent them while cutting. This made it hard to create a centered cut. More importantly, once we flattened the bent coin, the cut we were left with was not uniform. Near the center, the gap the cut created was very narrow but on the edge of the coin it was very wide. Therefore, when we tried to put these coins together, they did not align well or fit together nicely.

Thus, we had to search for a new method to cut through the coin, which is when we discovered the Dremel, and changed our making process!

Similarly, when creating the actual Sphericoin art, we noticed that the coins didn’t actually pick up a lot of paint when we painted the sides, giving us weak and faint lines like this:

Therefore, in order to make sharper lines with the coins, we decided to dip them in acrylic. Even after doing this though, we still had some trouble with the coin marks towards the end of their path through the page. Therefore, we decided to turn the page around so that we could get defined lines on both ends, as you can see in our final picture.

The Math Behind the Sphericoin

As we mentioned at the beginning of this post, the sphericons are a family of shapes that are obtained by “rotating a symmetric polygon about a mirror axis to get a solid of revolution, cutting that solid into two equal pieces, and then putting the pieces back together differently” (MOMATH).

The basic sphericon is defined by the American Mathematical Society as a “(double) cone with a twist”. Two right-angled cones can be taken and connected base to base. Then, if you imagine the points of this object as facing up and down, you can slice it vertically, and rotate it 90 degrees. With this rotation, the shape is now a sphericon.

The version that we created looks different because we used two flat pieces – coins. However, if you imagine a coin as a flattened cone, it is just a variation of the classic sphericon. If you look closely at the following image of a regular sphericon from Instructables, you can trace the edges that our hand-made Sphericoin had. Therefore, we still retained the integrity of the shape, and hence its meandering path.

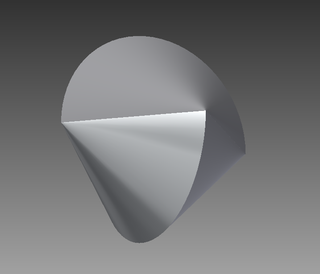
As this Action Labs video explains, the reason a sphericon meanders instead of rolls is because, when we do our polygon modification, we essentially create an object with one continuous surface. When an object rolls, the whole surface wants to be in contact with the ground. Therefore, for a sphericon to achieve this, the ground will essentially “trace” the shape of the sphericon’s surface, and therefore make it meander across the surface its on.

As we mentioned earlier, the sphericons are an entire family of “modified” symmetric polygons. Therefore, we could make a vast variety of sphericons that have different surface outlines from the basic sphericon – each of which would have a unique path. In this Maker’s Muse video we found a lot of interesting variants of sphericons that we could create. While the basic sphericon creates a squiggly path down a relatively straight line, other sphericons have paths that stop, turn, and go sideways where the classic sphericon might not, which gives rise to very intriguing path patterns. In future projects, we would love to explore the different paths that distinct sphericons make, and the mathematics defining these pathways!