By Jackson, King, and Jon

A Tangram set is a traditional Chinese 2D- puzzle consisting of seven flat pieces called tans, which are put together to form a square. The objective of the puzzle is to rearrange the tans into a particular shape (given only an outline or silhouette) using all seven pieces, which may not overlap. It is a popular mathematical game that encourages creativity and problem-solving while exploring concepts of geometry, symmetry, and spatial awareness. When the pieces are arranged together they suggest an amazing variety of forms, embodying many numerical and geometric concepts.

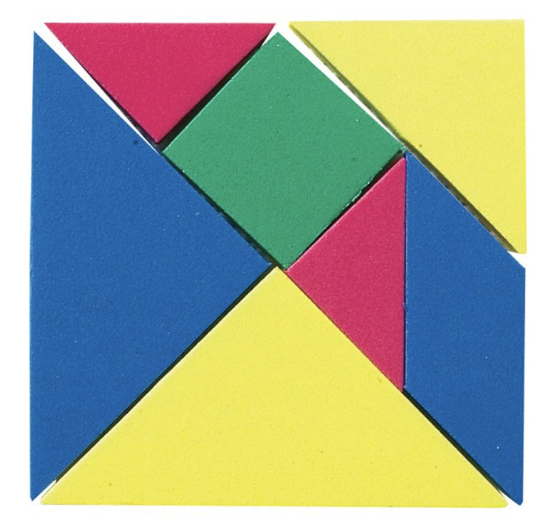
The mathematics of the tangram set is a fascinating exploration of geometry, symmetry, and spatial reasoning. Comprising seven specific pieces—five triangles of three different sizes, a square, and a parallelogram—this ancient puzzle encapsulates a myriad of mathematical principles. The three different-size Tangram triangles are all similar, right isosceles triangles. Thus, the triangles all have angles of 45°, 45°, and 90°, and the corresponding sides of these triangles are in proportion. Each piece, known as a tan, can be used to construct a perfect square, demonstrating the concept of area conservation despite the variation in shape. The tangram encourages an understanding of geometric transformations, including rotation, reflection, and translation, as players manipulate the pieces to form various shapes. It also offers a practical application of fractions and ratios, as the tans can be combined in ways that visually represent these concepts. Beyond its utility in teaching mathematical concepts, the tangram set challenges solvers to engage in problem-solving and logical reasoning, making it a timeless tool for mathematical exploration and creativity.

There are seven shaped pieces in a Tangram square, which can be creatively used to make various figures.

Tangram pieces are widely used to solve puzzles that require the making of a specified shape using all seven pieces. The three different-size Tangram triangles are all similar, right isosceles triangles. Thus, the triangles all have angles of 45°, 45°, and 90°, and the corresponding sides of these triangles are in proportion. Hence, it is easy to see that all the angles of the Tangram pieces are multiples of 45—that is, 45°, 90°, or 135°, and that the small Tangram triangle is the unit of measure that can be used to compare the areas of the Tangram pieces. Since the medium triangle, the square, and the parallelogram are each made up of two small Tangram triangles, they each have an area twice that of the small triangle. The large triangle is made up of four small Tangram triangles and thus has an area four times that of the small triangle and twice that of the other Tangram pieces. What is a Tangram set?

We chose to create a tangram set because it is an interactive game that promotes creativity and problem-solving. Our project investigates the Tangram’s mathematical foundation, looking at its utility in exploring geometric concepts. Having seven distinct shapes, the Tangram serves as a practical tool for demonstrating fundamental geometric concepts such as rotation, reflection, and tessellation. It promotes an understanding of Euclidean geometry, specifically in the context of congruence, similarity, and the Pythagorean theorem. By engaging with the Tangram, we directly apply these principles, enhancing our knowledge of spatial relationships and transformational geometry. This both enhances our mathematical understanding and passes time in a fun and creative way.

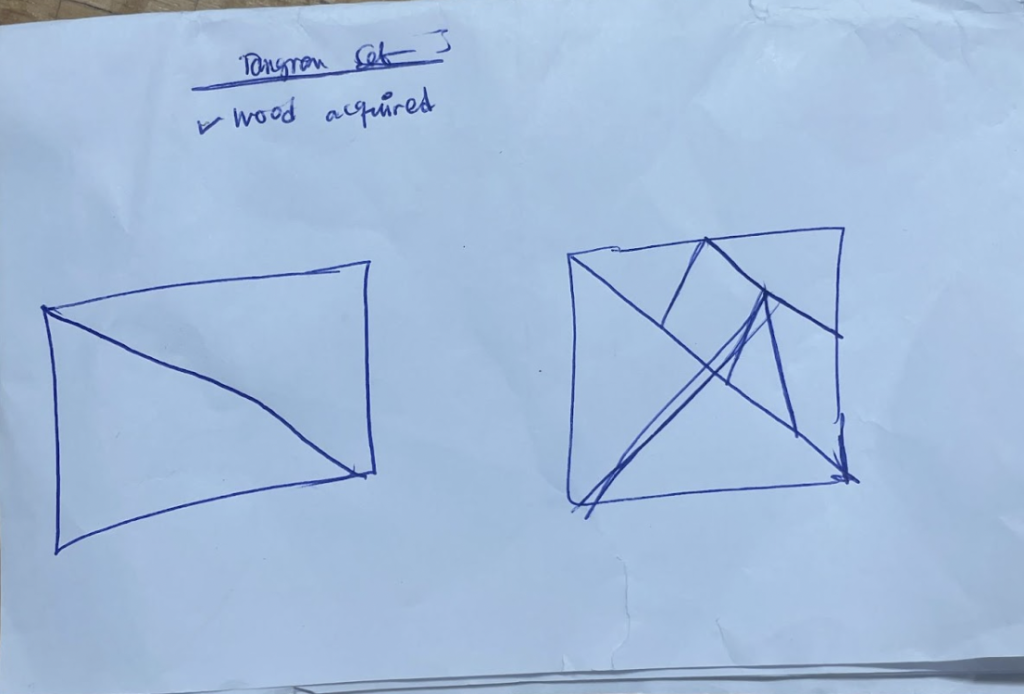
Our project exploration required that we make a Tangram set with wood, first in 2D and then try for it in 3D. 

Steps we took:

First, we selected a high-quality piece of wood from the woodshop that is very flat and free from imperfections to guarantee a smooth, uniform finish for the Tangram pieces. 

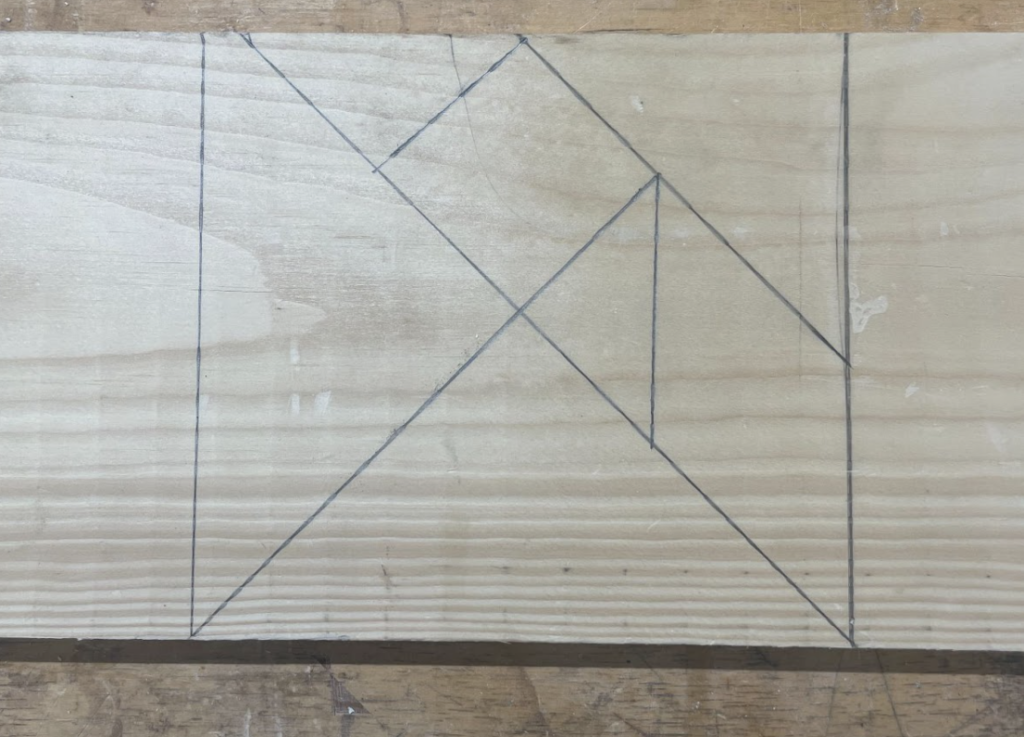
Next, using a scribe and a long ruler, we measured and marked the wood based on the geometric requirements of the Tangram shapes.

With careful hands, we then used a saw to cut along the marked lines, transforming the wood into the seven distinct shapes of the Tangram set. Following the cutting, we sanded the edges of each piece thoroughly, making them smooth and safe to touch.

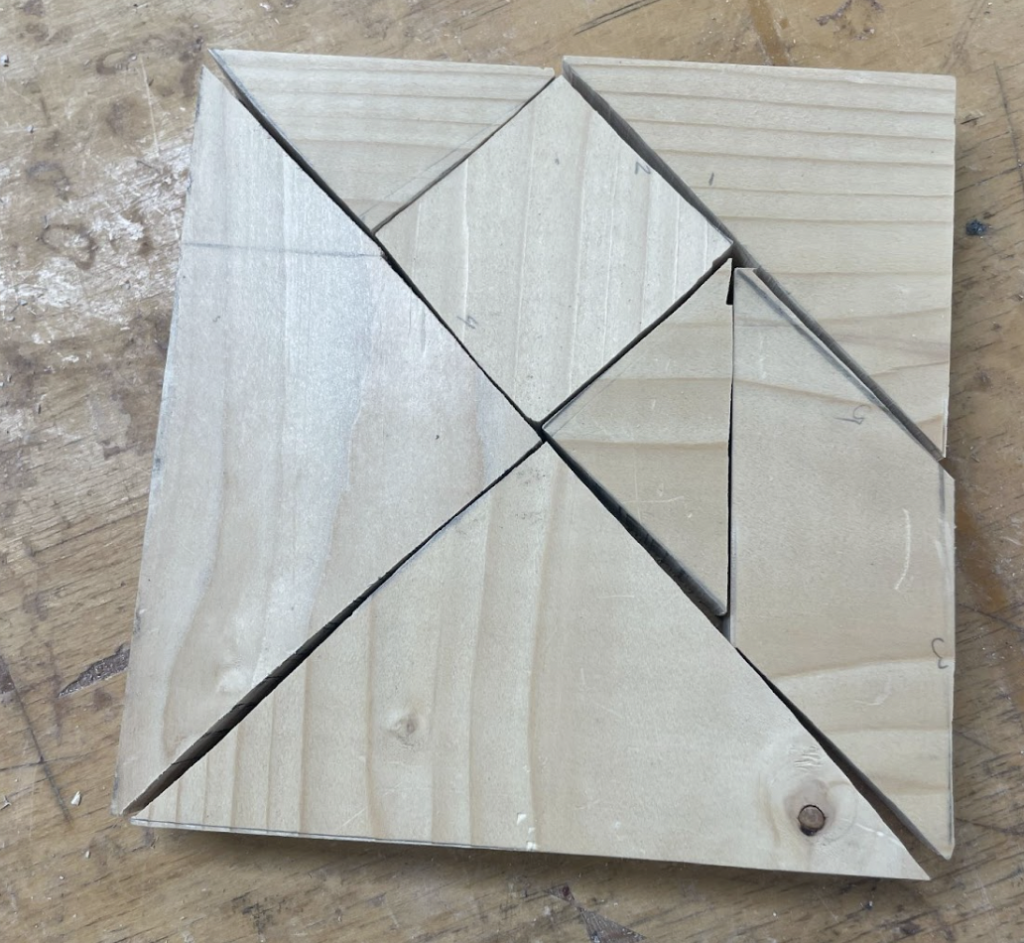
The final step in our process involves applying a finish to the wood, which not only protects the pieces but also brings out the natural beauty of the material, completing the transformation from a simple piece of wood into a set of Tangram puzzles ready for endless configurations.