Author: Jaanvi Chopra
-

Making with Jaanvi
Overview of the Semester As the semester wraps up, I wanted to compile all of my projects into one blog post and show a timeline of how the semester went. Project 0 – Kaleidoscopes and Stereographs For this project, I began by creating a kaleidoscope, then Kishore taught me how to make a stereograph. I…
-

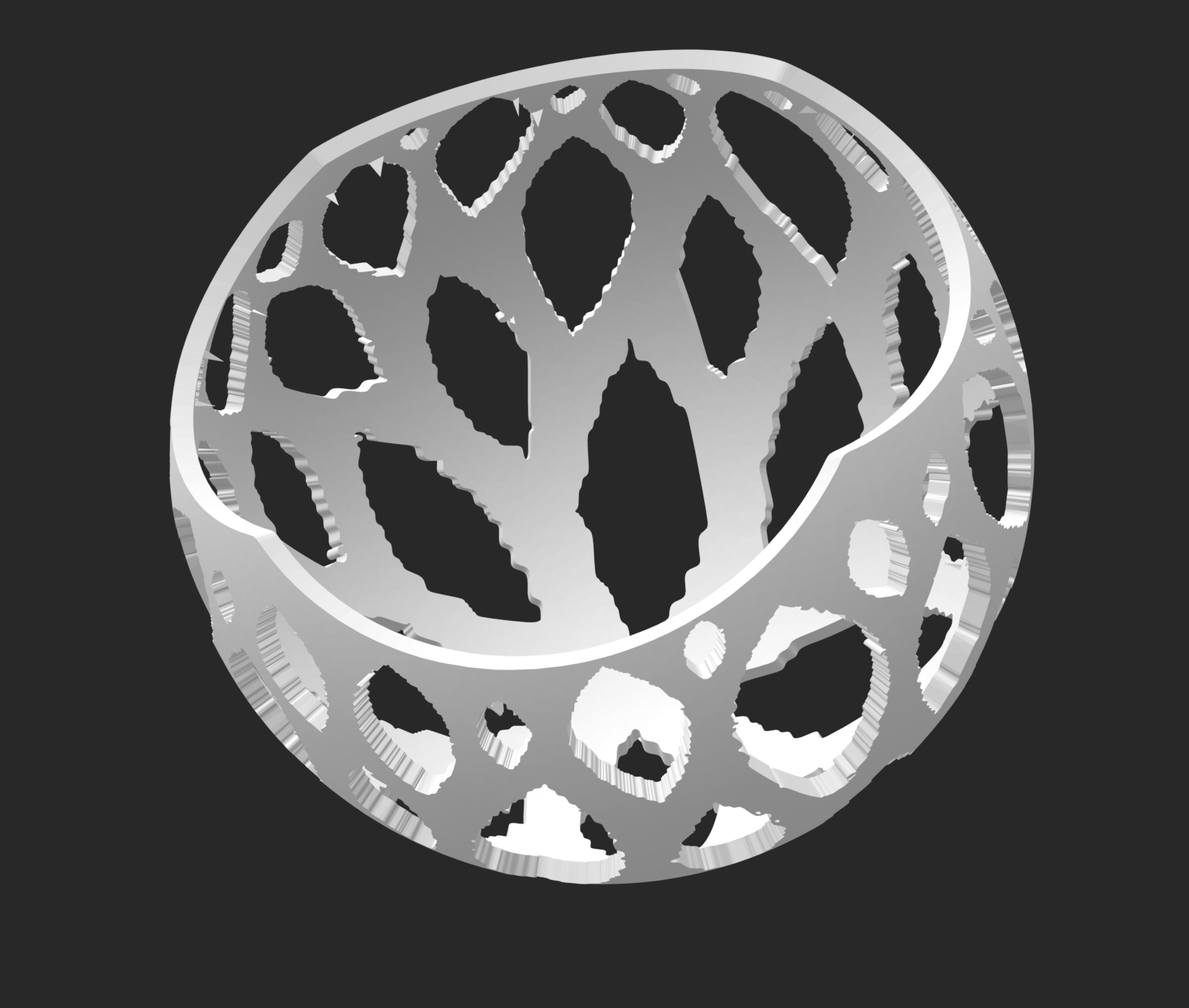
Mandalas, Continued
Project Overview Following my previous project about mandala art, I decided to explore the possibility of melding the concepts of three-dimensional structures with mandala art. This led to the inspiration of crafting a 3D octahedron, where each of its triangular faces serves as a mandala, each with unique symmetry groups and properties. Goal: Create an…
-

Tensegrity – An Experimentation
by Jaanvi Chopra and Kishore Rajesh What is Tensegrity? A tensegrity structure is usually made of two pieces, one which stays flat, and another which seemingly “floats” on top. The name comes from “tension” and “integrity.” Basically, the top part is supported by the tension in the center string/chain connecting it to the bottom part…
-

Group Theory and Mandalas!
I was inspired to study the properties of Mandalas because they are beautiful and intricate designs. Mandalas are artistic representations of the cosmos in both Hinduism and Buddhism. The very word “mandala,” with its roots in the ancient Sanskrit language, translates to “circle,” a shape that universally symbolizes harmony and unity. Week 1: Planning and…
-
Kaleidoscopes and Stereographs
Introduction Project 0.B gave us a great opportunity to learn about new technologies and mathematical concepts. We first recreated Kishore’s project, where we created Stereographic Projection Spheres. This project leveraged a mapping of a sphere onto a flat plane, and made use of 3D printers. Then, we recreated Jaanvi’s project by creating kaleidoscopes which represented…
-

Coxeter Groups Through the Lens of Kaleidoscopes
Making Kaleidoscope to Represent Coxeter Groups. What was our process with this project? The project began with the conceptualization phase, where we identified the goal of creating a kaleidoscope to represent the symmetries of Coxeter groups visually. This phase involved extensive research into Coxeter groups to understand their mathematical foundations and how these could be…
-

Some Ideas I am Excited About
One of the main reasons I found myself drawn to “Making Mathematics” was because I am interested in how mathematics can be represented in a creative manner. Going through the “Project Ideas” in our syllabus, I found a couple of ideas that I found interesting, which I will be discussing in this post. I was…